The CATO Principle, Part 2: An Origami Tsunami
By Matt Baker - Thursday, November 19, 2020
In Part 1 of this column, I talked about Bob Hummer's head-scratching CATO Principle. In this follow-up post, I'd like to share my favorite routine using the principle, John Bannon's "Degrees of Freedom" (from the book Dear Mr. Fantasy). [Thanks to John Bannon for giving me permission to explain my presentation and handling for his routine here.]
A Puzzling Diversion
We begin with a mathematical challenge from the 1920s by the famous English puzzle master Henry Ernest Dudeney which, historically speaking, was one of the inspirations for card tricks like "Degrees of Freedom". It appears as puzzle #367 in Dudeney's book "536 Puzzles and Curious Problems". The problem is this:
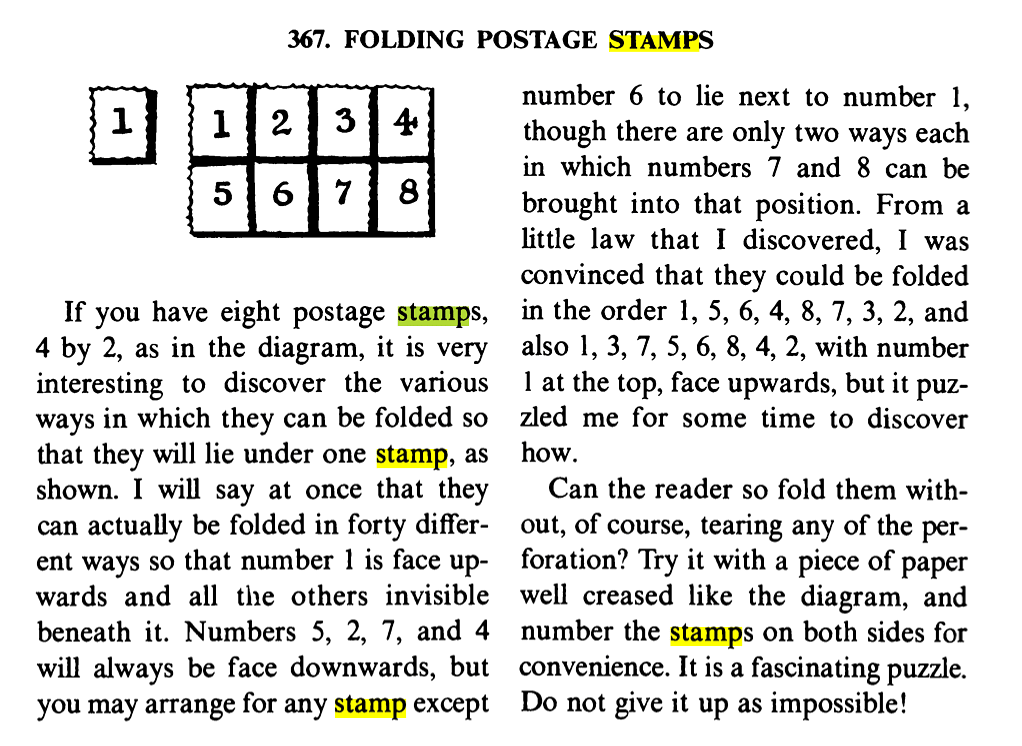
Dudeney's solution can be found at the end of this post.
Degrees of Freedom: Presentation
Now, on to "Degrees of Freedom". The trick is performed from a shuffled deck in use (RIP Paul Cummins). I like to talk about how the ability to control specific cards can be extremely valuable when cheating at poker, and I mention that there are many different ways to accomplish this, including sleight of hand and psychological influence. However, I tell the spectator, I'm unfortunately not at liberty to say which method I personally use. I offer to demonstrate and let the spectators determine for themselves what kind of surreptitious influence might be at work.
As you say this (or, even better, substitute your own script), go through the deck face-up and remove an assortment of cards, placing them face-up on the table. Make it look like you're choosing cards more or less at random, but in fact take out exactly 16 cards and make sure that among these cards are the 10, Jack, Queen, King, and Ace of Spades. (The other cards which are removed should include some other face cards and some other spades, but also some random spot cards which aren't spades. With just a little practice, this can be accomplished fairly quickly and nonchalantly from a shuffled deck.) Collect these 16 cards face-up and put the rest of the deck aside; it won't be used again.
(Phase 1) Give the packet a quick face-up overhand shuffle (or, better yet, let the spectator do it), saying that you'll first mix up the order of the cards. Next announce that you'll mix up the orientations as well. Take the cards two at a time, seemingly randomizing their face-up / face-down orientations as you do this, and hold each pair of cards above the table, asking, "Should I keep these as they are, or flip them?" Do as the spectator says and then drop the pair of cards on the table. Each time ask "Keep or flip?", as you create an apparently random pile on the table. There's a method to this random-looking madness, though, which I'll discuss in just a few moments.
(Phase 2) Pick up the jumbled pile of 16 cards and continue to offer the spectators choices, saying, "Let's take this just a little bit further." Spread over the first four cards, hold them above the table, and once again ask, "Keep or flip?" Do as the spectator instructs and then drop the packet to the table. Repeat with the next four cards, but allow the spectator to choose not only "keep or flip", but also whether the packet goes above or below the other cards on the table. Do the same thing with the other two groups of four. When you're finished, quickly ribbon spread the 16 cards to show their randomized condition and then scoop the cards back up.
(Phase 3) "I'm going to give you one more set of choices", you say. Deal the cards in a snake-like fashion into a 4x4 rectangular grid:

Explain that the spectator will now play a little "origami" game, folding the cards in on themselves until just a single pile remains. The spectator may choose any one of the four edges of the rectangle: top, bottom, left, or right. Whichever she chooses, "fold" the corresponding row or column of the grid onto the adjoining one, flipping the cards over in the process. Continue until a single packet remains.


To conclude, remind the spectator that she made all of the choices herself and say, "Now, regardless of whether I've been using psychological influence, sleight-of-hand, or some other type of deception, I think you'll agree that the end result is rather astonishing --- not to mention quite invaluable to a gambler." Ribbon spread the cards to show that all the cards are face-down except for five face-up cards, which form a Royal Flush in Spades.
Degrees of Freedom: How To
The trick is self-working, although you will need to memorize (and practice) three simple rules for "randomizing" the orientations of the cards as you take them out in pairs. Let's call the five cards which make up the Royal Flush our "target cards". As you remove each pair of cards from the face-up packet, remember the following rules:
- If they're both non-target cards, flip the top card so that they're face-to-face.
- If exactly one is a target card, keep both cards face-up but make sure the target card is on top.
- If they're both target cards, flip the bottom card so that they're back-to-back.
In each case, after you've made the necessary adjustment to the order and orientations, you can flip the pair over if you wish (it doesn't matter, so be spontaneous) before asking, "Keep or flip?" Practice this sequence until you can do it as nonchalantly as possible.
That's more or less the only part of trick which isn't self-explanatory (as far as the performance goes), but there's one more detail which the perceptive reader might have noticed. If you want to ensure that the final revelation consists of all face-down cards except for the Royal Flush, it might be necessary to reverse the packet before the final spread. To know whether or not such a reversal is needed, simply keep track of a single target card of your choice during the final "folding" sequence. (If you know whether this particular target card ends up face-up or face-down, you know the final orientation of all the target cards.)
I urge you to give this routine a try... you'll most likely fool yourself. Remarkably, the pattern does not reveal itself until the very end; at every stage of the trick prior to the denouement, the orientations of the cards seem completely random.
Degrees of Freedom: A Peek Under the Hood
And now for the million dollar question: why does this work? [Full disclosure: Vanishing Inc. unfortunately did not pay me a million dollars for this explanation.]
Well, first of all, the three "randomization" rules guarantee that, at the end of Phase 1, the cards will alternate face-down and face-up except for the target cards. In other words, if you were to reverse the orientations of the five target cards you'd find that the cards precisely alternate face-down and face-up.
To see why this happens, it suffices to consider each of the three basic rules separately.
Case 1: Suppose you have a pair of cards which are both non-target cards. If you orient them face-to-face, the top card will be face-down and the next card will be face-up. And if you flip these cards as a pair, this condition is maintained.
Case 2: Suppose exactly one of the cards is a target card. Orient them face-up, with the target card on top. Now, if you were to flip the target card over, the top card would be face-down and next card would be face-up, just as in Case 1.
Case 3: Suppose both of the cards are target cards. Orient them back to-back, so that the top card is face-up and the bottom card is face-down. If you were to reverse both target cards, the top card would be face-down and next card would be face-up, just as in Case 1.
That explains what happens in Phase 1. The CATO principle, as explained in my previous post, ensures that the cards still alternate face-down and face-up, with the exception of the target cards, at the end of Phase 2.
The "origami" sequence in Phase 3 has the ultimate effect of reversing the orientation of every other card. If you try it with the cards starting in alternating face-down/face-up order, you will begin to see why this is the case. No, really, do it: reverse the orientation of every other card in a face-down packet of 16 cards. Now deal the cards into a grid according to the snake pattern above. You will find that the grid now has a "checkerboard" pattern, with (non-diagonally) adjacent cards always having opposite orientations. Now choose a bordering edge of the rectangle and "fold" it over. What happens? Well, you'll notice that, because we started with a checkerboard pattern, the orientation of the cards within each two-card pile you just formed will match. Repeat with a different edge, and you will get the same result; continuing in this way, at each stage the cards within any given pile all have the same orientation. Inductive reasoning tells us, therefore, that at the end of the folding procedure we will have a single pile consisting of either all face-up or all face-down cards.
Of course, what I just described is not the situation in the actual trick. In the trick itself, the situation is the same as in the previous paragraph but with the target cards reversed. It follows that, at the end of Phase 3, we will have the same outcome as in the previous paragraph --- a single pile consisting of either all face-up or all face-down cards --- but with the target cards reversed. Et voilá.
A Simpler Version of the Origami Sequence
Here's a simpler version of Phase 3 which can be useful when you don't have enough table space for a 4x4 grid. Deal the cards from left to right into four piles. Now explain that you're going to "fold" the cards into a single pile and the spectator can choose which side to start from, left (gesture with your left hand) or right (gesture with your right). If she says "left", fold the leftmost packet to the right by flipping it over onto the adjacent packet. If she says "right", fold the rightmost packet to the left. Repeat two more times, each time offering the spectator a choice of "left" or "right". You will end up with a single packet, as in the grid version.
If you prefer the 4x4 grid (as I do), but for whatever reason don't like the snake pattern, other layout patterns work as well, for example a clockwise "spiral":
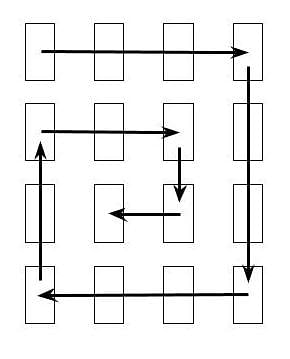
You can also experiment with other numbers of cards, like 12 or 20, instead of 16.
Credits
I've already mentioned Henry Ernest Dudeney and Bob Hummer as spiritual godfathers for this routine. Here are some other credits.
My handling of Phase 1 of "Degrees of Freedom", which is slightly different from John Bannon's, was influenced by a presentation that Art Benjamin showed me many years ago.
The identity of the person who first applied the Dudeney folding procedure to playing cards seems to be unknown, although some of the credit belongs to Martin Gardner for his pioneering article "Paradox Papers" (The Pallbearer's Review, Vol. 6, No. 9, July 1971). Richard Vollmer worked out a variation of Gardner's ideas in "Mr. Koenig's Tapestry" (Apocalypse, Vol. 14, March 1991, also published as "Mr. King's Tapestry" in Roberto Giobbi's book "Card College Lighter") which is perhaps the most relevant precursor to "Degrees of Freedom". Richard Vollmer was shown the Dudeneyesque folding procedure with playing cards by Jean-Yves Prost, who did not know the name of the person who had showed it to him.
The three simple rules which surreptitiously "set" the five target cards in Phase 1 are Bannon's variation on a Steve Freeman idea (further explored by Lennart Green). Steve Freeman made a number of important advances to this plot; for example, he appears to be the first to have a group of cards such as a Royal Flush appear as the climax of a CATO-style routine.

Kuniyasu Fujiawara published a routine called "Automatic Ace Triumph" (Genii, May 2000) which was subsequently described by Jim Steinmeyer in his book "Ensuing Impuzzibilities" and has taken off in popularity recently due to the fact that it can be done entirely over Zoom in the spectator's own hands. Adrien Lacroix marketed version of this routine called "Virtual Triumph", which was recently performed by Penn and Teller.
The underlying mathematics of "Automatic Ace Triumph" is closely related to the other routines discussed above, including "Degrees of Freedom". In the Genii write-up of "Automatic Ace Triumph", Richard Kaufman explicitly traces the roots of the routine back to Henry Dudeney and Bob Hummer.
Additional credits can be found in John Bannon's "Dear Mr. Fantasy".
A number of other routines making use of related ideas can be found in the following links: https://www.conjuringarchive.com/list/category/2124 and https://www.conjuringarchive.com/list/category/2747
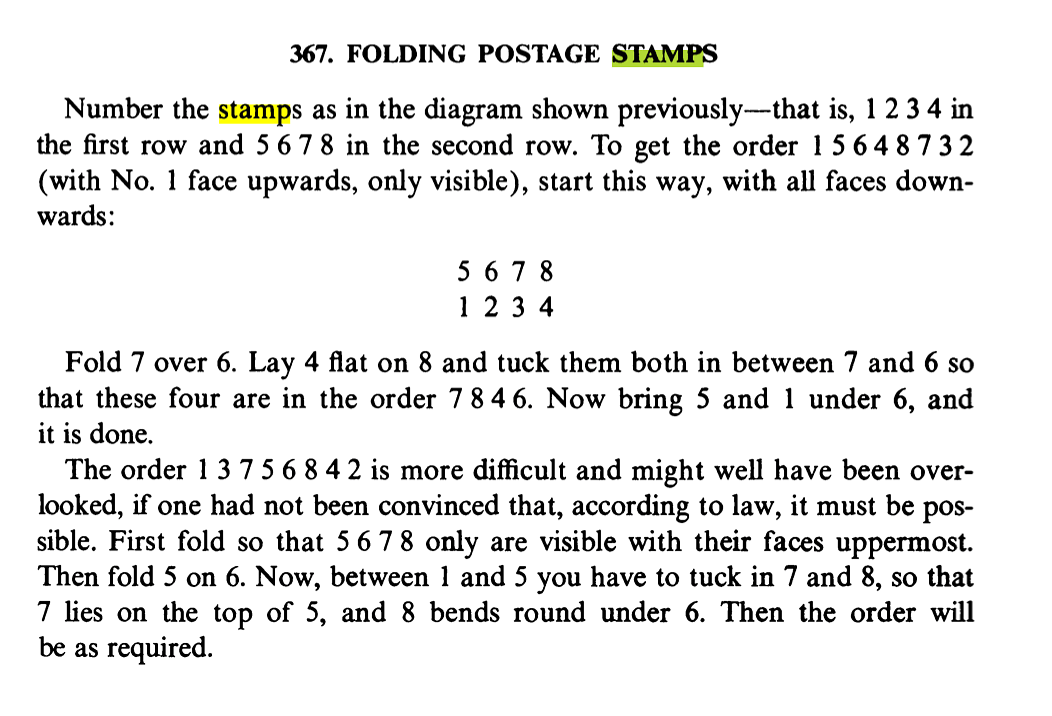
Postscript: Solution to Dudeney's Stamp Folding Puzzle
And finally: here, as promised, is the solution to Dudeney's folding postage stamps puzzle. I will confess that I have no idea what the "little law" is to which Dudeney refers. Perhaps one of my readers will rediscover it, and potentially inspire yet another great card trick!
Back to blog homepage
Similar posts on the blog:
